Domain Of Bessel Function Order 1

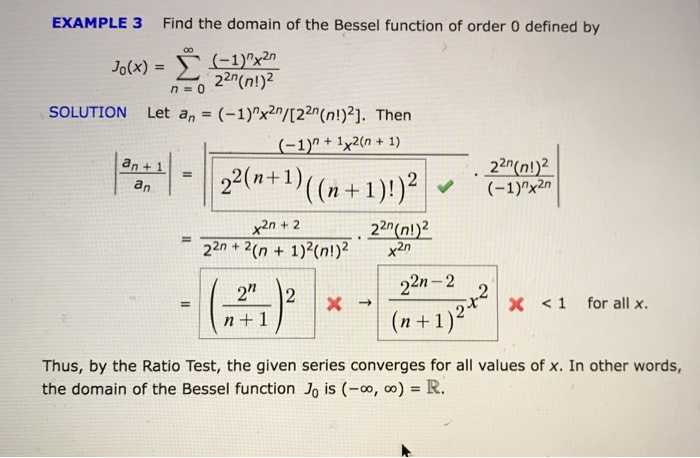
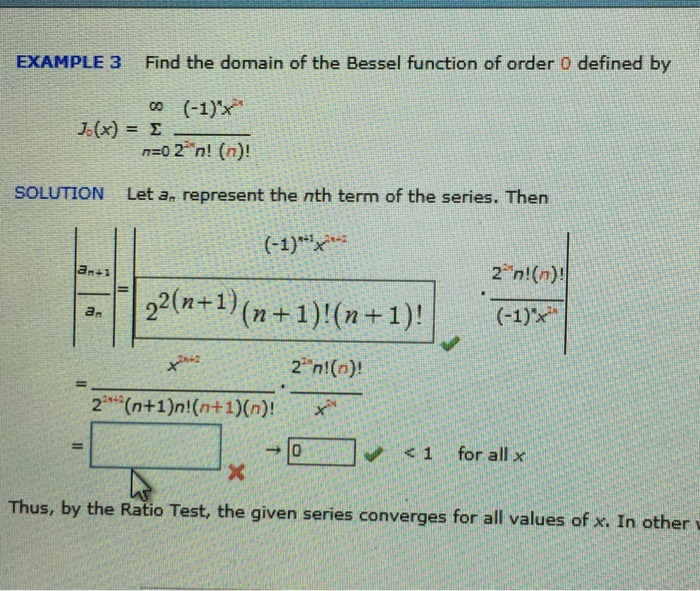
Then thus by the ratio test the given series converges for all values of x.

Domain of bessel function order 1. N 1. B graph the first several partial sums on a common screen. The analysis of bessel forms and their factorizations shows a variety of behaviors depending on the parameter m.

B 25 to this aim we multiply the power series of ezt 2 e z 2t and after some manipulation we get. Construction of a rst solution. Find the domain of the bessel function of order 1.

1 x 2 n 1 here the nth term for given series is un n. Bessel function of order one power series domain practiceconvergence of function. The generating function of the bessel functions of integer order.

Is called the bessel function of order 1. Although α and α produce the same differential equation it is conventional to define different bessel functions for these two values. The bessel functions of the rst kind j n z are simply re lated to the coe cients of the laurent expansion of the function w z t ez t 1 t 2 x 1 n 1 c n z tn.

1 13 note that h1 0 r is the domain of bessel forms for re m 0. The function j 1 defined by. In particular there is a kind of a phase transition at re m 0.

2x 0 1 2 2. Bessel s equation bessel s equation of order α with α 0 is the second order differential equation 1 x2y xy x2 α2 y 0 in order to find all solutions we need two independent solutions. Bessel functions first defined by the mathematician daniel bernoulli and then generalized by friedrich bessel are canonical solutions y x of bessel s differential equation for an arbitrary complex number α the order of the bessel function.