Domain Is Bounded Or Unbounded
Contributions Of The Domain Modes To The Bounded And Unbounded Domain Download Scientific Diagram
2 Comparison Of Bounded And Unbounded Domain Transfer Functions For A Download Scientific Diagram
What Is The Difference Between Closed And Bounded In Terms Of Domains Mathematics Stack Exchange
Bounded And Unbounded Interval Set Notation For Inequalities And Domain Gcse Precalculus Youtube
Unbounded Domain An Overview Sciencedirect Topics
Open Closed Domain Mathematics Stack Exchange
Any function that isn t bounded is unbounded.
Domain is bounded or unbounded. In mathematics a function f defined on some set x with real or complex values is called bounded if the set of its values is bounded in other words there exists a real number m such that for all x in x a function that is not bounded is said to be unbounded. If you can t the domain is unbounded. In general a bounded context is a boundary within which the ubiquitous language is consistent.
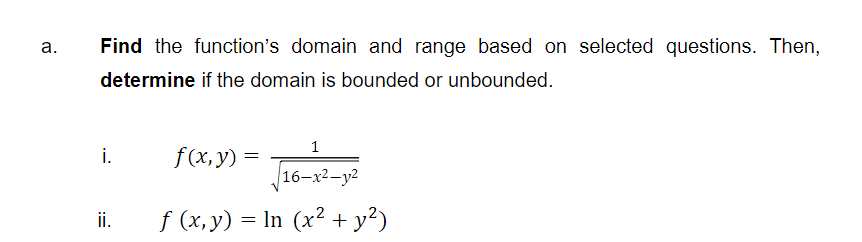
In this question the domain is unbounded because it continues forever in the first and thirds quadrants. For an insurance company the core domain is insurance a supporting domain could be client portfolio and a generic domain could be something like timesheets. In passing from a bounded to an unbounded domain we encounter certain difficulties in convergence which can be removed only by a change in the normalization process.
If f is real valued and f x a for all x in x then the function is said to be bounded from above by a. To figure out whether the domain is bounded or unbounded ask yourself if you could draw a circle on the graph of the domain that would contain all of it. A function can be bounded at one end and unbounded at another.
In today s lesson we explore what it means for sequences to be bounded or unbounded and also the relationship between bounded ness and convergence or divergence. Bounded context opens part iv strategic design. A region in the plane is bounded if it lies inside a disk of finite radius.
Chapter 2 talks in detail about how a domain is divided into bounded contexts and chapter 3 is the best source on drawing context maps. If the boundary points form a closed loop then the domain is said to be bounded otherwise they are considered unbounded. In contrast to bounded operators unbounded operators on a given space do not form an algebra nor even a linear space because each one is defined on its own domain.
This modified process was introduced by h. Answering quiz questions about sequences. Or books definition.
File Bounded And Unbounded Functions Svg Wikipedia
Bounded Function Unbounded Definition Examples Calculus How To
Telecommunication Systems 1 Ppt Download
Illustration Of 1 Unbounded Extrapolation And 2 Bounded Extrapolation Download Scientific Diagram
Can Unbounded Discontinuous Functions Be Locally Bounded Mathematics Stack Exchange
Solved 1 Consider The Function Defined As R 1 Cos X Y Chegg Com
Metric Glossary
Solved Given The Functonfxyin 6y 5x Answer The Follo Chegg Com
Bounded Exercises
Solved A Find The Function S Domain And Range Based On S Chegg Com
Solved A Choose The Correct Domain 1 O A All Points In Chegg Com
4 A Find The Function S Domain B Find The Function S Range C Find The Boundary Of
Math Tutor Functions Theory Elementary Functions
