Domain And Range From The Graph Of A Continuous Function Brackets
You can also use restrictions on the range of a function and any defined parameter.
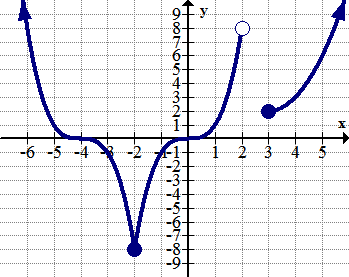
Domain and range from the graph of a continuous function brackets. When looking at a graph the domain is all the values of the graph from left to right. This line is a function. The line and function to the left has a domain and range of all real numbers because as the arrows indicate the graph goes on forever both negatively and positively.
Because the domain refers to the set of possible input values the domain of a graph consists of all the input values shown on the x axis. X x values or inputs of a function and the range is all. Definition of the domain and range.
We will draw the graph of the function using the graphing calculator. The range is all the values of the graph from down to up. It would pass the vertical line test.
The domain and range are all real numbers because at some point the x and y values will be every real number. Another way to identify the domain and range of functions is by using graphs. To limit the domain or range x or y values of a graph you can add the restriction to the end of your equation in curly brackets.
Y y values or outputs of a function. In a continuous graph to determine the range you should focus on looking bottom to top of the graph. Another way to identify the domain and range of functions is by using graphs.
We use interval notation to help us describe the domain and range for graphs that represent continuous situations. The range is the. The domain is all.